You may have encountered polyominoes at some point in your life, because they are used in puzzles popular for more than 100 years, including ones like Tetris.
A polyomino is a plane geometric figure formed by joining one or more equal squares, edge to edge. The name was devised by Solomon Golomb in 1953 in a now-classic textbook, and then popularized by Martin Gardner in 1960; see the “Mathematical Games” column in Scientific American.
Today we’ll play with our updated LEDAS logo, which we released a year ago. As you can see, it consists of four pentominoes. A pentomino is a polyomino of order 5, i.e. 5 equal-sized squares connected edge-to-edge.
Splitting the LEDAS Logo Into Polyominos
Our question today is this: what is the number of ways in which to cover this area – a 6х6 square, and missing a 2х2 corner by dividing the inner area made of 12 white cells?

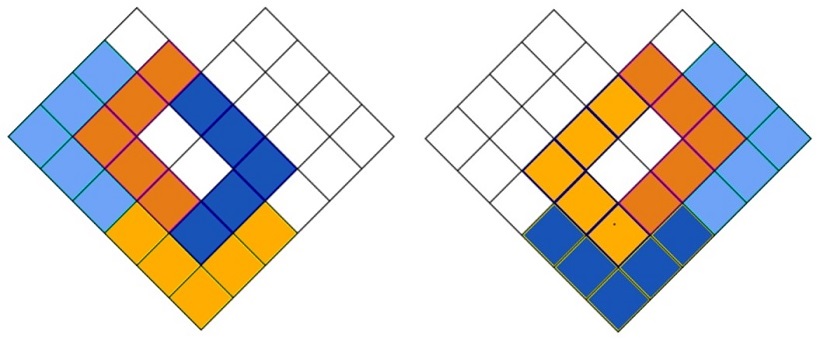
Let's agree ahead of time that two configurations are considered the same if they are just made by replacing colors or mirroring the image. For instance, the following two configurations are the same, because we simply repainted a pair of pentominoes and then mirrored the picture:
But what about the white areas in the configuration? As you can see, they make up three parts of the initial 12-square polyomino. Moreover, there are at least two ways to split them:
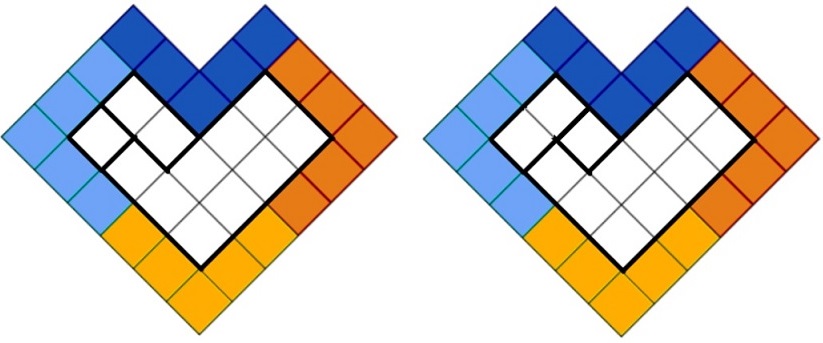
We have found a way to split the 12-square white figure into three pieces, and then pack all four initial pentominoes and three white polyominoes into the logo area (the square 6х6 missing the 2х2 corner). This means that there are at least three ways to pack the seven polyominoes into the logo’s area. But is there another way? And how many ways are there?
The Goal of the LEDAS Polyomino Contest
The goal is to find the best splitting of the white 12-square figure on N pieces. What do we mean by best? It means that we want to have a maximum number of ways to pack everything back into the logo’s area.
For each found configuration, you get +1 point. But for each additional splitting of the white figure, you lose 7 points.
This means that if you find M different configurations after splitting the white figure into N pieces, you have M – 7 * N points. The goal is to maximize your points.
In our example, N=3 and M=3, so the resulting score is 3 – 3 * 7 = – 18.
Reporting Solutions
We invite readers to submit their original solutions. Send your configurations to quiz@ledas.com. In your email, please include the explanation and the expected number of points.
We will post the names of all who submit a correct solution. (If you don't want your name made public, then please say so in your submission.) We look forward to seeing what you can come up with!
The Table of Results
1. Sergey (6144 – 7 * 7 = 6095) — 8-Jan-2021
2. Daria (1538 – 5 * 7 = 1503) — 22-Dec-2020
3. Ivan (448 – 4 * 7 = 420) — 21-Dec-2020
4. Ivan (256 – 4 * 7 = 228) — 17-Dec-2020
5. Alex (208 – 4 * 7 = 180) — 9-Dec-2020
6. Demo (3 – 3 * 7 = –18) — 3-Dec-2020